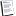
1.8% is the probability of 3 wins from 5 races with the wins occurring in a specific order, ie, one of the 10 possibilities I pointed out previously, not just any 3 wins from 5 races for which the prob is 10*0.35*0.35*0.35*0.65*0.65=18.1%.

There is only one way to get 3 wins from 3 races and that is WWW and this has a probability of 1*0.35*0.35*0.35=4.9%. Hence it is much more difficult to get 3 wins from 3 races than 3 wins from 5 races.

For 5 races where W represents the probability of a win in one particular race and L represents the probability of a loss in that same race the following terms give the probabilities of the number of wins shown below

0 wins 1*L^5
1 win 5*w^1*L^4
2 wins 10*W^2*L^3
3 wins 10*W^3*L^2 (this gives 0.181 or 18.1%)
4 wins 5*W^2*L^3
5 wins 1*W^5

You should be able to see the pattern for W and L. The numbers 1 5 10 10 5 1 come from Pascal's Triangle, which is too difficult to format here but you can look it up. The sum of all the individual probabilities is always 1 for a particular number of races.

For 3 trials the numbers are 1 3 3 1 and the calculations are

0 wins 1*L^3
1 win 3*W^1*L^2
2 win 3*W^2*L^1
3 win 1*W^3 (this gives 3 wins from 3 races)

As an illustration take the top ranked place percent horse which Knowles claims has a 21.7% (say 22%) win rate (though I dispute this figure for Saturday racing.) Suppose you want the probability of at least 1 win from 5 races using this factor. You could add the probs for 1, 2, 3, 4, 5 wins or simply take the probability of all losses away from 1.

Therefore W=0.22 and L=0.78 and Prob of at least one win from 5 races = 1- prob of all losing = 1-0.78^5 = 0.71=71%

Similarly, the probability of at least 1 win from 3 races = 1-0.78^3=0.52=52%.

This is very important maths for punting so I hope this helps even if you use a spreadsheet. (Not that I have the holy grail)

gunny